The Rafter Square
Laying out a roof with this basic tool and a new generation of accessories.
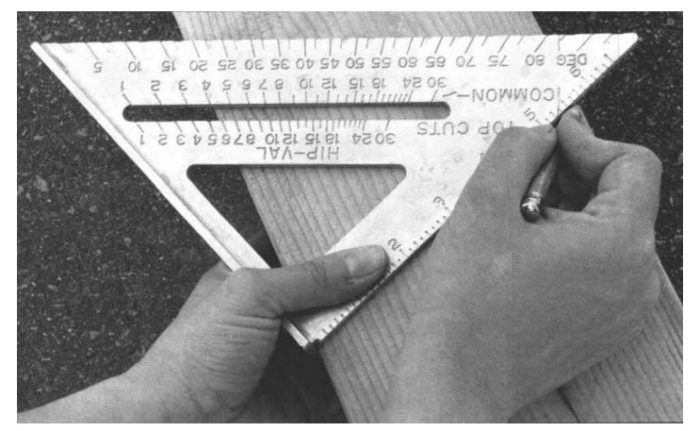
Synopsis: Here is a guide to using the rafter square for more than squaring up a board. The author outlines various calculations that can be carried out with tables printed on the square. Sidebars describe the Speed Square and a tool called the Squangle. A chart explains how to use a calculator for roof framing.
Few carpenters would neglect to include a steel square when packing their toolboxes for a job. Yet, when pressed, quite a few good builders will abashedly admit that they generally use the square just for scribing a cut-off line on stock too large for their combination square. The steel square can serve a variety of functions, from stairbuilding to making simple checks for right angles, but it’s especially useful for laying out rafters and other roof-frame members. With a little instruction, anyone can lay out cuts for common rafters, valleys, hips, jacks and gable ends. This doesn’t require a knowledge of trigonometry, just a simple understanding of the geometry involved.
The rafter square
This versatile tool consists of two parts — the body, or blade, and the tongue. These two meet at the heel. The body is 24 in. long and 2 in. wide, and usually represents the level line, or run, in laying out rafters. Plumb, or rise, is represented by the tongue, which is 16 in. long and 1 1/2 in. wide. The face and back of the square are usually imprinted with edge scales and math tables. The latter distinguish a rafter square from a framing square.
Squares are made of steel, usually painted black, or aluminum. Aluminum squares are typically more expensive, but lighter and less liable to bring second-degree burns to your palms on hot summer days. The best squares have their numbers stamped deeply into the metal rather than painted on. Most of them come with instruction books that are a handy toolbox reference.
The use of the rafter square is based on the geometry of right triangles. All right triangles have a 90° angle, which can be used to describe the intersection of a plumb and level line — the intersection of the tongue and the body of a framing square. Right triangles also share another quality: when the rise and run of a right triangle are increased proportionally, the hypotenuse lengthens proportionally, too, although its slope or pitch remains constant. A stair with a rise of 6 11/16 in. and a 10-in. tread has the same pitch as an 8-in-12 roof because the proportion between rise and run for each of them is the same. Since rafters are nothing more than the sloping side of the triangle, the rafter square acts as an infinitely expanding intersection of plumb and level, allowing you to use the rafter stock as the hypotenuse. More information on how the proportional nature of the rafter square can come in handy will be presented later.
A useful quality of right triangles, discovered by Pythagoras, is that the sum of the square of the sides equals the square of the hypotenuse (a2 + b2 = c2). To the roof framer this means that if the rise and run are known, then the length of the rafter can be easily calculated.
For more photos, drawings, and details, click the View PDF button below:
Fine Homebuilding Recommended Products
Fine Homebuilding receives a commission for items purchased through links on this site, including Amazon Associates and other affiliate advertising programs.

Magoog Tall Stair Gauges

Speed Square

Metabo HPT Impact Driver (WH18DBDL2)