I thought this question had just been asked and answered recently, but I couldn’t seem to find the thread, so excuse the redundant post if so:
I’m trying to figure out how to join an arc to a 90 degree square. I’m saying it’s trim because I’ll need to do the same thing for trim later on a different project, but here it is actually a border and a railing for a curved deck.
I drew up a couple sketches to try and help explain the problem, but I’ll try to put it in words as well:
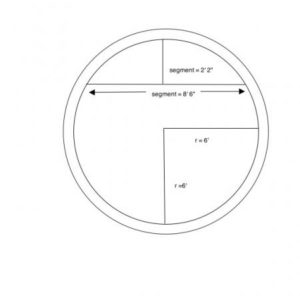
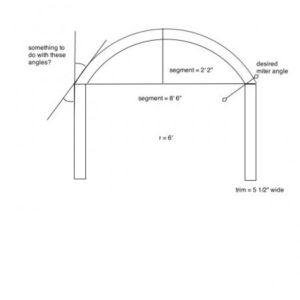
I scribed a 6′ radius for the curbed front of a deck. The arced section is only 8’6″ wide (this is rough, can’t remember the exact dimension now that I’m away from the jobsite), and say 2′ 2″ high. The deck board is 5 1/2″ wide.
So when I glue up my curved piece, if I were to cut it straight across to butt against the straight deck board going at 90 degrees, the width of the cut along the curved piece would actually be longer than 5 1/2″ since it is coming in at an angle because it’s an arc and not a full half-circle. So I would want to miter the two pieces together by finding the angle at which the arced section comes in at the straight section. But therein lies the math that I have no idea about.
Here’s the couple of sketches that I drew up to illustrate (hopefully) what I’m trying to make into words.
So if anyone can decipher that and help me to understand what equation I need in order to figure this out I would be so very happy.



















Replies
bump
Without doing the math, the simpleist way, after you make the arch, is to lay the straight deck board over it and mark the points where the two intersect on each side of the board. That will be the angle you have drawn in the second sketch.
Right, that makes sense.
So that will work well for flat stock, like the deck boards.
But now I'm still holding out for some sort of equation that will yield me at least close numbers since the next job where I would use it will be a bunch of custom millwork with a more complex profile. Even though I'll be able to sneak up on it by holding the trim over the other, I'd like to think I could use the magic of math to get me there with less cutting an re-cutting.Even though I liked math back in high school, I also liked girls and music. I can remember the girls and the music that I liked, but I can't seem to remember the math!
Thanks for the help,Miters turned out great.Paul
If the arched board is the same width (measured along a radius) as the uprights, you'll need to cut the uprights square. If the widths are unequal, the method suggested above (lay one over the other and mark) is the easiest solution.
Mike Hennessy
Pittsburgh, PA
Everything fits, until you put glue on it.
That's true if you have a complete half-circle. He's drawn a shorter arc segment, though.So Dave's technique is the way to go: mark both edges of each board where the edges cross each other, then connect the dots on both boards to draw the cut lines.AitchKay
"That's true if you have a complete half-circle. He's drawn a shorter arc segment, though."
Looking at pic 2 again -- you're right.Mike HennessyPittsburgh, PAEverything fits, until you put glue on it.
Are you looking for a Hunting Miter?
I searched the 'net using "join curved and straight molding hunting miter" ... The illustrations at the bottom of this page, Chest of Books, Windows.Part 10, look interesting.
That's a cool article.Mike HennessyPittsburgh, PAEverything fits, until you put glue on it.
Woah....my head's about to explode but I think you've started me on the right track. I'll spend some time seeing if I can decipher and apply. It looks like that article you sent might be just the right thing.Math is awesome.Paul
BTW thanks for searching the web for me as well. It always amazes me that whatever thing you wan to do, there's always a way to do it. It's just finding that way that is the tricky part. At least it never gets boring!
Hipaul, the math isn't too tough.
The hunting miter can be determined using only geometry. The curve is always an equal distance from a point ... the focus, which is the center of the circular molding ... and a line, the directrix. This definition of a parabola goes back to the geometers of classical Greek civilization.
The relative positions of the circular molding and straight molding govern the formula:
y = ±x²/(4p)
The value of p (easily determined from the drawing) is the distance from the vertex of the parabola to either the focus or the directrix.
If you get stuck when you move on to your next project involving profiled stock, post your sizes and measurements (preferable an image like you did in this thread) and I'll try to talk you through the math.
Joe Bartok
Thanks Joe,I might take you up on the help at some point. It always helps me to be able to talk it through with someone the first time so that I really understand each step. If I can really understand each component step, then I'll be able to fully grasp the how and why of the whole.I'm on a math quest now to re-learn all about chords and arcs and trigonometry. I've got my work cut out for me.Paul
Thanks for the help with the hunting miters. I'm still trying to get some time to sit down and work through the math so that I understand it, but haven't had the time yet.
I had just picked up a book on circular/curved carpentry, but haven't had time to look through that yet either.
But the overlap measuring worked just fine for this application. I'll probably be back in touch when I get to the profiled trim.Paul
hipaul,
This link might be helpful as it has some pictures of the layout.
http://picasaweb.google.com/josephfusco.jr/ArchedWindowTrim#
http://www.constructionforumsonline.com
What DaveRicheson said.
That's how I solve this type of miter angle problem - but I am mathmatically "challenged".
Jim